 Dialectic Chaos
I recommend you read the page "An Application" in the "Wise Virgins" section of the
"Metamath" area of the site before this section, else things will make little sense.
Clearly using the dialectic we would expect it possible that in any four-colourable map, shrinking all regions of one particular colour to a "point" or "empty set" would result in a three colour plane graph, also connected as a tripartite map. Is this correct?
The "horse" of operation (A∩B)∪(A∩C) when A is the empty set results not in the union of B and C, but the empty set itself. However, the complement ((A∩B)∪(A∩C))c results in the whole set space.
The horses "rider" is missing in both cases.
We deduce then that the rider would be the fourth set "D" riding B and C whilst "A is empty".
Then if D rides B and C the result is that in a (three-colourable) tripartite map with regions having the same colour represented by "A" sent to "empty" sets, our "meet" is valid as follows.
D = (b + c) = (B v C)c = Bc ∩ Cc
Because the only set left is the elements coloured with "D", we see a remarkable but unwarranted similarity between the Klein four group operation and this dialectic system.
Where D, B and C represent the sets of all regions filled by similar colours unique to each of B, C and D.
And therefore the rider D may in all readiness represent D ∪ (Bc ∩ Cc)
Yet this is equal to Dc ∩ (B ∪ C)c.
However the appearance may be, that the "meet" operation is exactly the same as K4 subgroup addition in the octal, it must clearly be pointed out that Dc in no way equals (B ∪ C)c. I.e. because "A" is not empty to begin with!
However, that said - is it possible that every four-colourable graph with a set of regions in one colour removed is also a tripartite (three-colourable) graph?
If this operation is satan's cause for assuming that there is some question over God's unique sovereignty, we may happily deduce that we could construct one rather than be fooled too easily!

Now, this graph may only be coloured one way - The blue regions must share the same colour. If blue is shrunk to the empty set, then yellow could indeed border itself. If a series of interconnected graphs as above, where every outer island becomes the centre of a new graph (i.e. yellow becomes nested as blue) then there is no colour that may be removed, such as,....
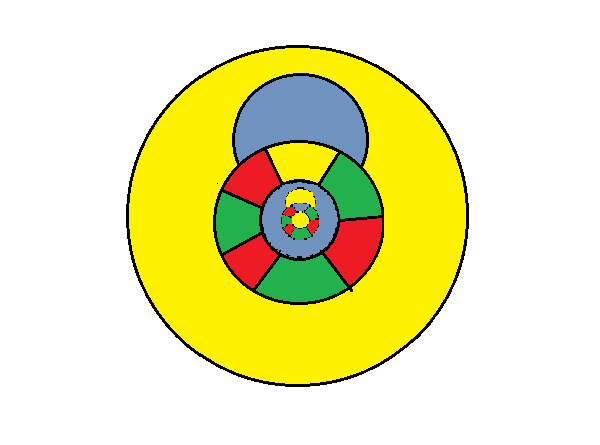
Now, I have only nested this graph once within itself. Simply continue the process a further two times using the four-colour map principle with outer regions in the red and green regions in the map above and proof done - the dialectic method has no basis in fact (it has a specific counter example to the contrary) - its own set theory is inconsistent!
However if the regions in blue in the first graph are shrunk in a manner where the edges of it's borders are shrunk to zero length then we do end up with a three-colourable map.
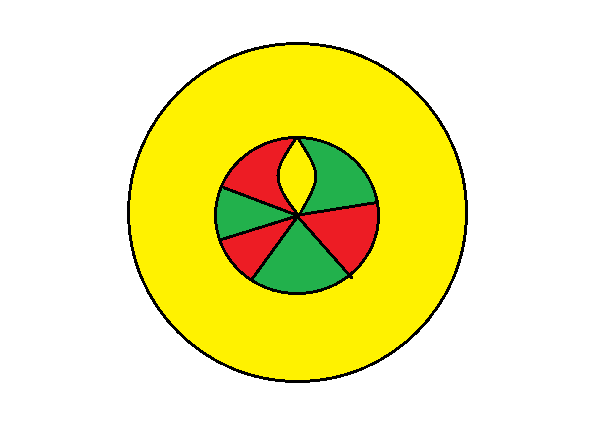
However the text indicates that the octal is in view: In effect we have each region separate - and into one blue region we may substitute the colour of a neighbour and delete the edge; In that manner the "yellow horse" posited rides out "conquering and to conquer" (Although this is not the reason for this phrase in the text.) . We see that one colour (blue) is "conquered" by yellow riding out conquering and now borders itself, and we have lost information because now two neighbouring regions have the same colour: the two bordering yellow regions remaining are then set for the horseman "to conquer",
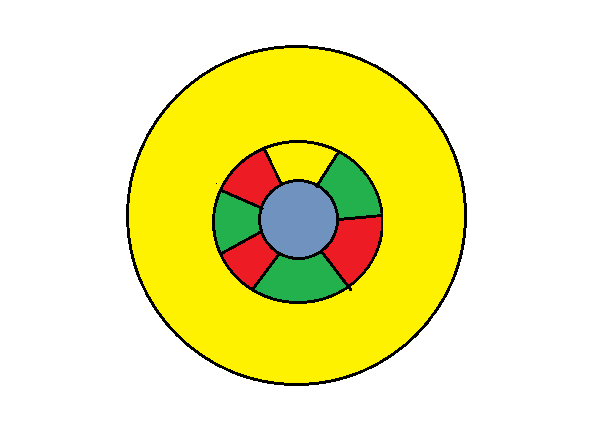
So since the text states bluntly that the empty set may not be exchanged for an element of an octal we see substitution supplies no answer either: (In the octal which is the correct underlying set theory) There is no "truth" in assuming one element to be empty (ignoring a "fact" to suit one's self) If we extend this substitution of yellow for blue for all blue regions then we obtain a three-colourable graph but neither of the two alteration methods above make the graph a "subgraph" of our original. (We have lost too much information) and we deduce that the dialectic method has no basis. The octal reduces to the K4 group with the substitution of one colour for another, yet we obtain a solution of the symmetry found in GF(4) rather than GF(8) - but there is still no morphism between them. (There is no embedding of the altered in the original.)
Continue To Next Page
Return To Section Start
Return To Previous Page |